Crumpled
January 15, 2012 at 3:28 PM by Dr. Drang
I found a link to this article in New Scientist the other day, and it’s been driving me crazy. The article is entitled “Scrunch time: The peculiar physics of crumpled paper” and it’s crap from start to finish.
The article—unsigned, and for good reason—focuses on the work of two physicists at the University of Massachussetts Amherst, professor Narayanan Menon and graduate student Anne Dominique Cambou. I’m always suspicious when physicists try to do structural mechanics, but the awfulness of the article was beyond my imagination.
It starts out badly.
How can a sheet of paper become an unaccountably tough projectile simply by the act of crushing? The answer might seem simple, but it turned out that finding a sound explanation required complex instruments and a lot of brain power.
Actually, the answer is simple. Thin flat sheets are stiff in resisting in-plane deformation (this is often called membrane action) and flexible in resisting out-of-plane deformation (bending). When you put a 90° crease in a sheet, what’s out-of-plane on one side of the crease is in-plane for the other; therefore, the two sides reinforce each other in the immediate vicinity of the crease.
This isn’t new or novel. Cold-formed steel and aluminum sections are thin sheets of metal that have been bent into shapes that provide the stiffness necessary to use them as structural supports.
(image lifted from rebelsteel.com.au)
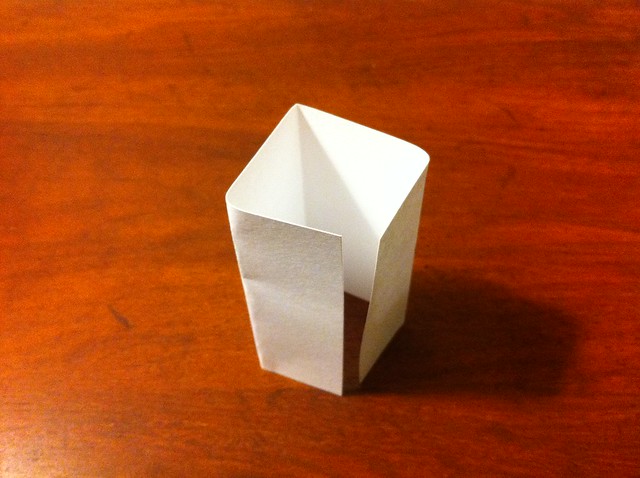
It works with paper, too. Here’s a 3×5 index card folded three times into a sort of square cross-section.
The folds stiffen the card enough to support the weight of a book.
You could do the same thing with a sheet of paper, but it would take more folding because the paper is thinner. The buckling strength of a flat sheet is a function of its width/thickness ratio1—in a folded sheet, the width is defined as the distance between adjacent creases. As that ratio goes up, the buckling strength goes down. So for a thinner sheet, you need narrower distances between the creases to get the same strength. Hence, more folding.
In this example, the load is coming from one direction, so I can get the strength I need by making all the creases parallel. But if I were to set the folded card on its side, the book would squash it flat. To get strength and stiffness in every direction, you need creases in every direction, which is what crumpling paper into a ball does. Conceptually, at least, this is pretty simple stuff.
There’s an analog in fiber-reinforced plastics. Some FRPs have all the fibers aligned in one direction, which gives them great strength and stiffness in that direction, but in that direction only. Some FRPs use chopped fibers scattered higgledy-piggledy; this doesn’t give them great strength in any particular direction, but gives them decent strength in all directions.
Update 1/15/12
I should have used this opportunity to link to one of my favorite posts here: an explanation of pull-top cans that includes a brief discussion of using sheet metal folding to change the flexibility of different parts of a structure.
The article gets worse:
Despite their insubstantial constitution, wadded paper balls are capable of feats of considerable strength. They are the ultimate packing material, for instance, able to support and cushion objects far heavier than themselves. That’s unexpected, given their lack of internal buttressing. A house, by contrast, has supporting structures such as beams built into the architecture to explain why it is so rigid. “This is not stiffness you have designed into the ball,” says Menon. “You’ve just crushed it.”
No no no no no no! The crumpled ball has internal buttressing. That’s what all those creases are. And while the creases may not be designed (in the sense that you don’t specify the position or orientation of any them), there’s no question but that they’re there, giving the ball strength and stiffness. It’s just like the chopped fiber FRP. You don’t prescribe the position of any of the individual fibers, but by mixing them in the resin, you get internal reinforcement of the plastic.
The absolute nadir of the article comes in these three paragraphs:
The image yielded answers immediately. The first thing the researchers noticed were the ridges throughout the insides of the ball. They are the paper’s strongest points, and what fortifies them is a quality that you might not expect from paper.
It might rip easily, but it is very robust in one particular way, says Tom Witten, a solid-state physicist at the University of Chicago. To demonstrate, he picks up a flat piece of paper and tries to stretch it until it rips. It is really difficult to do.
This is tensile strength. It also manifests when you fold paper and thereby impart tension at the resulting crease. Like these creases, the ridges inside the paper ball hold the energy you imparted by folding the paper. They are also the reason that a paper ball cannot be compressed beyond around 90 per cent air without superhuman effort. Through a combination of the rigidity of the ridges and the energy they concentrate, they prop up the structure as well as any deliberate design.
Jesus H. Christ! Who wouldn’t know that there are ridges (i.e., creases) inside a crumpled ball of paper? What the hell did they think was in there?
And yes, paper has pretty good tensile strength. The pulp and paper industry relies on it to make rolls of paper. There are standards and specialized equipment for testing it. Newspapers and magazines couldn’t be printed without it.
As for the third paragraph, no, the creases don’t “hold the energy you imparted by folding the paper.” The energy you imparted went mostly into breaking the paper fibers and creating microscopic tears at the folds. The energy isn’t being “held,” it’s lost forever in the damage. If the energy were being held, the crumpled sheet would spring back to a flat shape when you let go of it.
It was this “hold the energy” nonsense that made me wonder how well the article represented the work of Menon and Cambou. While I can believe that physicists would ignore the years of research done by structural engineers, I couldn’t believe they’d screw up the fundamentals of energy storage and dissipation.
So I started Googling and soon came upon this article in Wired by Brandon Keim. Keim’s article is so much better, I could hardly believe it was describing the same work. Menon and Cambou sound much smarter when a smart reporter is describing their research. They’re no longer amazed by the stiffness of crumpled paper; they’re trying to work out the exact processes by which the paper gets into that stiffer form.
“If you’re not talking about simulation, but mathematical understanding of these things, that’s one step harder,” said Menon. “We understand the underlying equations of the mechanics of a thin sheet very well. Those have been around for a century. But solving those equations, to produce a physical understanding, is difficult even in simple cases. If you’re talking about a structure that owes its properties to 1,000 or more of these structures, interacting in complicated ways, that’s asking more than we can do now.”
After reading Wired, I came to understand that all the stupidity in the New Scientist article came from the article’s writer, not the scientists. That a science writer gets things wrong isn’t surprising. I actually expect it. But to get every single thing wrong—and there are mistakes in almost every paragraph of the article—is stunning.
-
Note for readers with a structural engineering background: Why am I not accounting for the length of the sheet? Because for this geometry the capacity is governed by local buckling, not overall column buckling. ↩