Obscuring my location more than necessary
July 24, 2024 at 3:06 PM by Dr. Drang
In yesterday’s post, I obscured my location by covering up the latitude and longitude after the decimal point.

How good is this? How big is the area that could be covered by these truncated coordinates?
Well, you could cheat by using, say, ArcGIS to create a map of the Chicago area bounded by lines of latitude (parallels) at 41°N and 42°N and lines of longitude (meridians) at 88°W and 89°W. Like this:
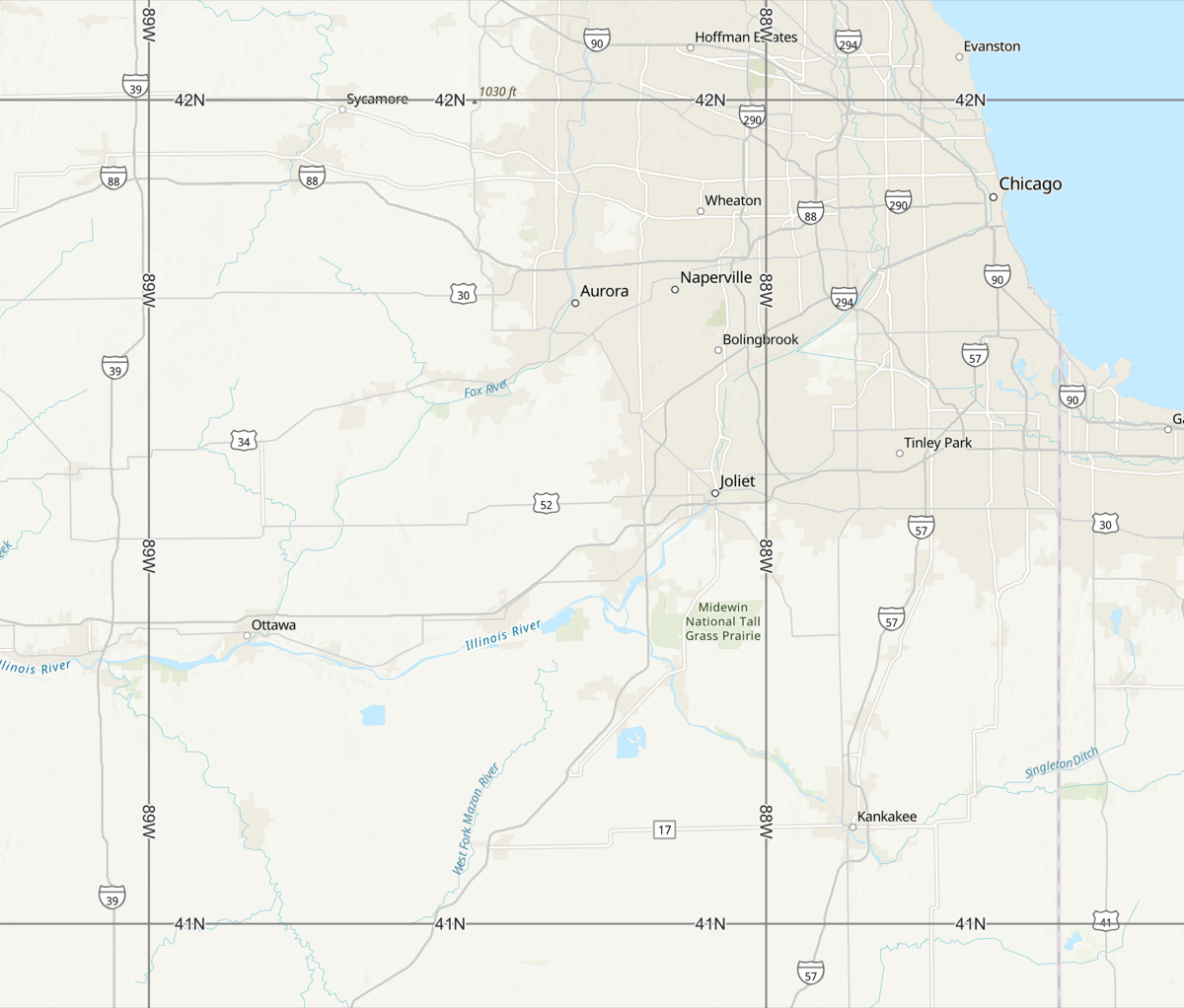
That’s obviously a pretty big area, and ArcGIS has measuring tools so you can get the lengths of the parallels and meridians. But you don’t need all this fancy stuff; you can get an estimate much more quickly with some simple calculations in your head or on a calculator.
The primary thing to know is that a nautical mile was originally defined as the distance on the Earth’s surface along one minute of arc. One degree is sixty minutes, so a very quick (and rough) estimate of the area would be a square that’s 60 miles on each side.
For some purposes—like my desire to obscure my location—this estimate is good enough. I’ve never made a secret of the fact that I live in Naperville, so I’ve already given up a more precise indication of my location than a 60-mile by 60-mile square, even if those two 60s are way off.
But if you need a significantly better estimate, you can calculate one with just a little more effort. The two biggest sources of error in the calculation above are:
- Nautical miles aren’t statute miles, they’re about 15% longer.
- Meridians converge as you get further from the equator. The convergence goes with the cosine of the latitude.
So our 1° long meridian is about 69 miles long. That takes care of the north-south distance. To get a better estimate of the east-west distance, we can multiply that 69 miles by the cosine of the average latitude,
to get 51.7 miles. This is why a 1° by 1° “square” looks more like a rectangle on a map.
I first thought I’d leave the first decimal place visible in the latitude and longitude figures. That would give me a rectangle of uncertainty of about 5 by 7 miles—good enough for my purpose. But as I was drawing the little checkerboard areas on the Tot screenshot, I found it much easier to use the decimal points as the left edges.
