Watermelons and soil
August 2, 2024 at 10:48 AM by Dr. Drang
I meant to write this post a couple of weeks ago, right after Presh Talwalkar published this video:
It’s the only mathematical brain teaser I’ve run into for which being a civil engineer made the solution a snap. I solved it in my head almost immediately.
Normally, this is where I’d encourage you to watch the video, but 10+ minutes is too long for this puzzle. You don’t need to hear Talwalkar go through incorrect and inefficient solutions. Here’s the problem statement:
I carried 100 kg of watermelons in the summer by train. In the beginning, their water content was 99%. By the time I reached the destination, the water content had dropped to 98%. In the end, how much did the watermelons weigh?
The key to solving it is to focus not on the mass and percentage of water in the watermelons, but on the mass and percentage of solid material. Why? Because the mass of solid material doesn’t change during the drying process. Only water evaporates away.
So we start with 1 kg of solid material (1% of 100 kg). We also end with 1 kg of solid material, but now it’s 2% of the total mass. And 2% of what is 1 kg? 50 kg. Done.
What does this have to do with civil engineering? Most civil engineers have to take at least one soil mechanics course (I took two and there was also a lot of soil mechanics in a pavement design class), and one of the first things you learn in such a class is how to quickly and accurately do calculations regarding the relative amounts of air, water, and solid material in soil. You need this ability because certain aspects of soil behavior—things like drainage, consolidation, and bearing capacity—depend to a large extent on these quantities.
A common technique is to use what’s called a block diagram to visualize the amounts and assist in the calculations. Here’s a generic block diagram from the book used in my first soil mechanics class, Introductory Soil Mechanics and Foundations by George F. Sowers.
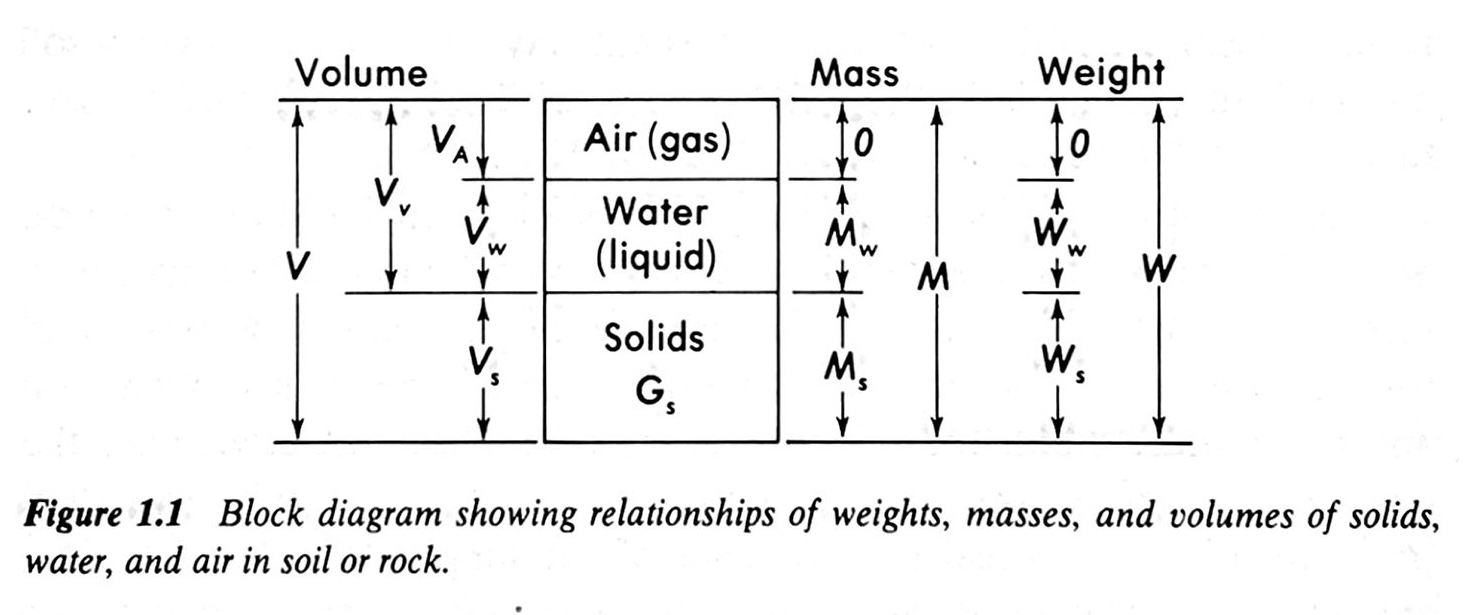
(Note that this is Figure 1.1 in the book. I told you it was one of the first things you learn.)
Volume figures are kept on one side of the diagram and mass or weight figures are kept on the other. Certain quantities are always known:
- The weight of air is zero.
- The volume of air in saturated soil is zero.
- The mass and volume of water in oven-dried soil are both zero.
Why would you put soil in an oven to dry it? To insure that when you then weigh the soil, all you’re weighing is the solid material—all of the water has been driven off. You then use this information along with the weight of the sample before it was dried to figure out how much water was in the soil originally. Do these sorts of calculations enough times and you won’t be fooled by a watermelon problem.
I did say, though, that I solved the watermelon problem almost immediately. The “almost” part also comes from my soil mechanics classes.
Look back at the problem statement and you’ll see that the 99% and 98% figures are called the water content of the watermelons. And we took the water content to be mass of the water divided by the total mass, which is a perfectly normal way to think of water content. But in soil mechanics, water content has a specific and different definition. It’s the mass of the water divided by the mass of the solids, not the total mass. My best understanding is that it’s done this way because there are advantages to keeping the denominator constant. While the amounts of air and water can change with the seasons and with loading, a given amount of soil will always have the same amount of solids.
So as I read the problem, I remembered the soil mechanics definition of water content, and it made me hesitate. Could they be using that definition? No, I decided, that would be silly, and I went on to solve the problem.
Suppose, though, that we were supposed to use the soil mechanics definition of water content. What would be the final weight in that case? I leave that as an exercise for the reader.
