And now it’s all this

I just said what I said and it was wrong
Or was taken wrong

Next post Previous post

Hyperloop

August 19, 2013 at 8:35 AM by Dr. Drang

The Hyperloop is a futuristic, high-speed transportation system dreamed up by Elon Musk, of PayPal, SpaceX, and Tesla Motors fame. The idea is that you and a couple dozen other travelers get into a capsule that’s loaded into a pneumatic tube and whooshed away at several hundred miles per hour. It sounds like Victorian-era science fiction, but we’re told that Musk has checked it out and found that it’s workable and both faster and cheaper than any high speed rail system.

I’m no billionaire industrialist, and I don’t pretend to know anything about the Kantrowitz limit, but I do know about some of the topics touched on in the Hyperloop proposal, and since I’m paying for this blog, I might as well use it.

Tube design

Let’s get this one out of the way quickly: I have nothing of substance to say about the structural design of the Hyperloop tubes because there isn’t enough information in the proposal to pass judgement. This was disappointing.

It’s true that the tube diameters and wall thicknesses are given, as is the average distance between the supports. Normally, this would be enough to make some quick calculations and test the design’s feasibility. In this case, however—probably because Musk¹ is concerned about buckling of the relatively thin-walled tubes—there’s a bit more to the tubes’ design:

In order to keep cost to a minimum, a uniform thickness steel tube reinforced with stringers was selected as the material of choice for the inner diameter tube. Tube sections would be pre-fabricated and installed between pillar supports spaced 100 ft (30 m) on average, varying slightly depending on location. This relatively short span allows keeping tube material cost and deflection to a minimum.

It’s the “reinforced with stringers” part that’s the problem. These stringers (“stiffeners” would be the more common structural engineering term²) are mentioned nowhere else in the proposal and no details of their size, shape, or spacing are given. You don’t see them in any of the drawings, either.

I have done some simple calculations assuming the tubes aren’t stiffened, and the stresses aren’t alarming, so I have no reason to believe that Musk can’t come up with a stiffened tube that’ll work.³

Thermal expansion

When I realized that the Hyperloop was intended to be a nearly straight, 300+ mile length of tube, my first question was “How are they going to handle the thermal expansion?”

In some piping systems, the expansion is dealt with by adding expansion loops or U’s like this spaced at appropriate intervals.

Source: Dynaflow Research Group

The idea is to use the pipe’s relative flexibility in bending (sometimes enhanced by the inclusion of special joints) to accommodate the expansion that builds up in long straight runs.

Another technique was used on the Trans-Alaska Pipeline, where the entire line is run in a serpentine fashion to achieve the same effect.

Neither of these is possible for the Hyperloop because the high speed of the capsule zooming along inside it means the pipe curves have to be very gentle. How, I wondered, is Musk going to solve the thermal expansion problem?

The answer turned out to be simple: he didn’t. There’s some hand waving and, possibly, a complete misunderstanding of how thermal expansion acts, but no actual solution. Here’s what the proposal says:

Earthquakes and Expansion Joints

A ground based high speed rail system is susceptible to Earthquakes and needs frequent expansion joints to deal with thermal expansion/contraction and subtle, large scale land movement.

By building a system on pylons, where the tube is not rigidly fixed at any point, you can dramatically mitigate Earthquake risk and avoid the need for expansion joints. Tucked away inside each pylon, you could place two adjustable lateral (XY) dampers and one vertical (Z) damper.

These would absorb the small length changes between pylons due to thermal changes, as well as long form subtle height changes. As land slowly settles to a new position over time, the damper neutral position can be adjusted accordingly. A telescoping tube, similar to the boxy ones used to access airplanes at airports would be needed at the end stations to address the cumulative length change of the tube.

OK, so the plan is to accomodate the thermal expansion of over 300 miles of pipe with some tube-in-tube slip joints at either end. Let’s do a few calculations to see how this works. It’ll be fun.

You may remember from physics that the thermal expansion and contraction of solids is governed by something called the coefficient of thermal expansion. Not the most imaginative name, I’ll grant you, but it has the virtue of being direct. For steel, the coefficient of thermal expansion is about $6.5\times {10}^{-6}\mathrm{°}{\mathrm{F}}^{-1}$. Don’t be thrown by the weird unit (reciprocal degrees Fahrenheit?); it makes perfect sense because we calculate the amount of expansion through this equation:

$$\Delta L=L\alpha \Delta T$$

where $L$ is the original length, $\Delta L$ is the change in length, $\Delta T$ is the change in temperature, and $\alpha$ is the coefficient of thermal expansion. The reciprocal degrees of $\alpha$ cancel with the degrees of $\Delta T$, and we get units of length on both sides of the equation.

I’m not an expert on the climate of California, but I’ll bet you have to assume a temperature swing of 100°F over the life of the Hyperloop (that’s the difference between the record low and record high in Fresno, for example). So, with a 300 mile (1,584,000 foot) pipe, the variation in length due to thermal expansion and contraction will be

$$\Delta L=(1,584,000\mathrm{f}\mathrm{t})(6.5\times {10}^{-6}\mathrm{°}{\mathrm{F}}^{-1})(100\mathrm{°}\mathrm{F})=1030\mathrm{f}\mathrm{t}$$

That’s right, a thousand feet of difference between the shortest and longest lengths of the pipe. That’s a 500-foot slip joint at each end of the pipe.

But the real problem isn’t with the slip joints at the ends; it’s with the pillar supports along the way. Musk thinks the dampers, “tucked away inside each pylon,” need to accommodate only “the small length changes between pylons.” Wrong.

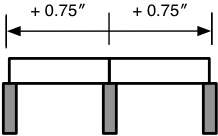
Imagine 200′ of pipe, supported on three pillars—one pillar in the middle and one at either end. If we increase the temperature 100°, the pipe will lengthen about 1½″. For this case, the dampers in the two end pillars have to accomodate ¾″ of movement, which seems doable.

Here, I’m showing the pipe sitting on the pillars at the low temperature. The lines and arrows above the pipe are where it expands to at the high temperature.

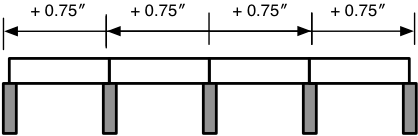
Now add 100′ of pipe and another piller to either end. How much movement do the dampers in these new end pillars have to accomodate? Just ¾″? No. They have to accommodate 1½″ because their lengths change ¾″ and their “starting points,” so to speak, also change ¾″.

Can you see where this is going? If so, you’re doing better than Musk. Eventually, the dampers in the pillars won’t be able to handle the movement because it’ll be greater than the pillar width. Eventually, the movement will be greater even than the distance between the pillars.

One solution to this would be to have many slip joints along the tube. That would also eliminate the need for the absurdly long joints at the terminals. But when I read what Musk says about slip joints,

Specially designed slip joints at stations will be able to take any tube length variance due to thermal expansion. This is an ideal location for the thermal expansion joints as the speed is much lower nearby the stations. It thus allows the tube to be smooth and welded along the high speed gliding middle section.

it seems like he doesn’t think his capsules will be able ride over them at high speed.

Another possibility would be to control the temperature of the tube so tightly that thermal expansion and contraction just doesn’t occur. I have no idea how to do this in a fault-tolerant way.

Finite element analysis

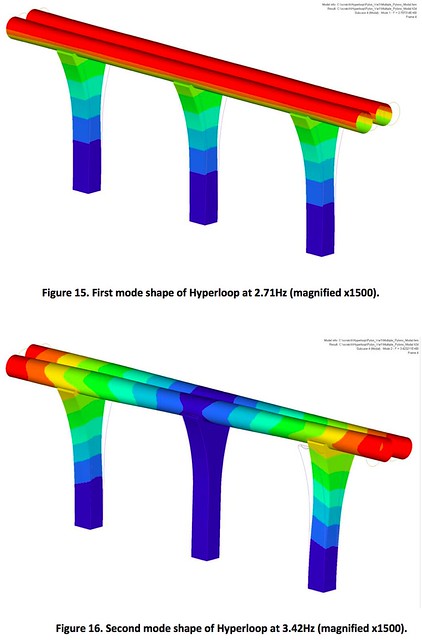
This is my favorite part of the proposal because it’s complete bullshit. It starts with these pretty pictures of the first and second vibration mode shapes of a section of the Hyperloop, as calculated through finite element analysis:

In these diagrams, the color coding is for deflection or movement: blue means less deflection, red means more. If you look carefully, you can see an outline of the undeflected shape. (Normally, I’d make fun of someone who thinks it’s necessary to mention that their mode shape diagrams are “magnified,” since mode shapes, by definition, don’t have a magnitude, but I’m going to let that go.)

According to the text of the proposal, these diagrams came from “[s]tructural simulations [that] have demonstrated the capability of the Hyperloop.” You might assume from the text, then, that what we see here is just a small section of a much bigger analysis. I doubt it. I’m pretty sure Musk analyzed just this little three-pillar chunk. Why do I think so? First, because to make a finite element model of the entire length at this level of detail would take an enormous amount of work and would almost certainly be beyond the capability of the software he’s using. Second, because the mode shapes shown here are what I would expect the mode shapes of a three-pillar system to be: one where the pipes swing back and forth more or less as a unit; and one where the two ends woggle out of phase with each other.

So what’s the value of getting the vibration mode shapes of this little section of the Hyperloop? Simple: it makes pretty pictures you can put in your proposal.

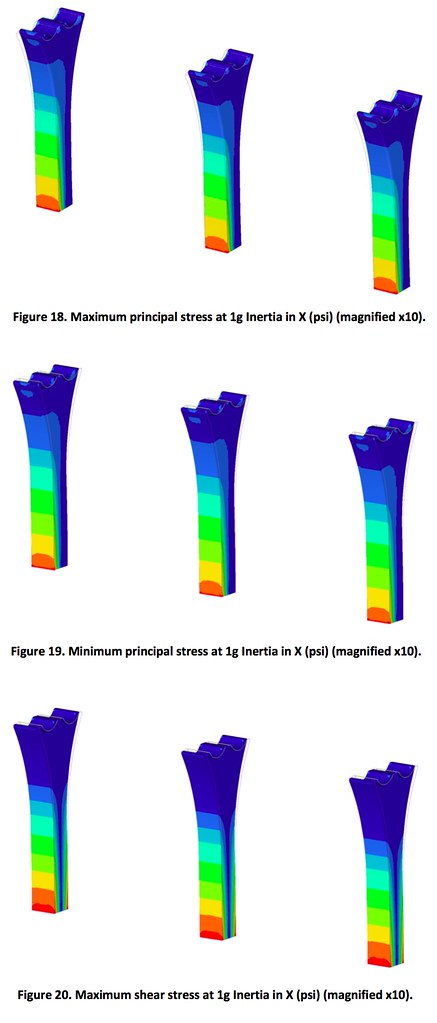
The stress contours are even more entertaining if you understand anything about the structural design of reinforced concrete. Here, Musk applies a 1 g lateral acceleration to his three-pillar model and plots out various stresses in the pillars. Now the colors represent stress instead of deflection, with blue being low stress and red being high.

Sarcasm fails me. No structural engineer of my acquaintance would do anything like this because

1. Each pillar is acting like a cantilever beam in flexure. Everyone learns how to analyze this in a strength of materials course in their sophomore or junior year of college; everyone knows the formulas by heart; everyone can do this problem in their sleep. There is no need for finite element analysis.
2. The results given in these diagrams, the principle stresses and the maximum shear stress, are of absolutely no value in the design of reinforced concrete in flexure. Even if you were stupid enough to build a finite element model just to tell you how a cantilever beam behaves, you wouldn’t ask for this output if you were trying to build it out of reinforced concrete.

Nothing in this section of the proposal has “demonstrated the capability of the Hyperloop,” nor was it intended to. This is not simply an error. This is eyewash for the rubes, the surest sign you’re dealing with a snake oil salesman.

Looping back

At the end of his proposal, Musk declares it to be “open sourced” and invites any and all interested to join him in his Hyperloop quest. I will politely decline his invitation.

I’m not saying that the problems with Hyperloop can’t be solved. Money, time, and talent can solve any problem that doesn’t involve breaking physical laws, but I wouldn’t put my money, time, or talent in the hands of someone who takes me for a fool.

Next post Previous post

Site search

Meta

• drdrang at leancrew
• Blog archive
• RSS feed
• JSON feed
• Mastodon
• GitHub repositories

Recent posts

Credits

This work is licensed under a Creative Commons Attribution-Share Alike 3.0 Unported License.

© 2005–2023, Dr. Drang