Minnesota jewel box bank
August 28, 2025 at 10:02 PM by Dr. Drang
Today’s Louis Sullivan jewel box bank is the National Farmers’ Bank in Owatonna, Minnesota. I stopped here on the way up to the Twin Cities, where I’ll be visiting my daughter and going to the Minnesota State Fair.

This bank was designed and built about a decade before the one I saw yesterday in Columbus, Wisconsin. That one was the last jewel box Sullivan designed; this is the first one. The park across the street from the bank has sign with a brief history of the building.

The semicircular stained glass windows are similar to what we saw in Columbus, Wisconsin, but here there are two large windows on adjacent sides of the building.1 The exterior as a whole is more ornate, largely because of the color used in the terracotta pieces.


Inside, the large semicircles are repeated on the other sides of the building, but these are filled with murals instead of windows.

You can see part of the other mural behind the clock that faces you as you walk in the front door. The clock is above the teller area; there’s a mezzanine behind it.

From the mezzanine is great view of the front stained glass window, the enormous hanging light fixtures, and the decorative piece above the entrance.

A “B” motif can be found in many of the decorative elements. They’re a nod to the bank’s president, Carl Bennett, who commissioned Sullivan at a time when he was having trouble getting work.

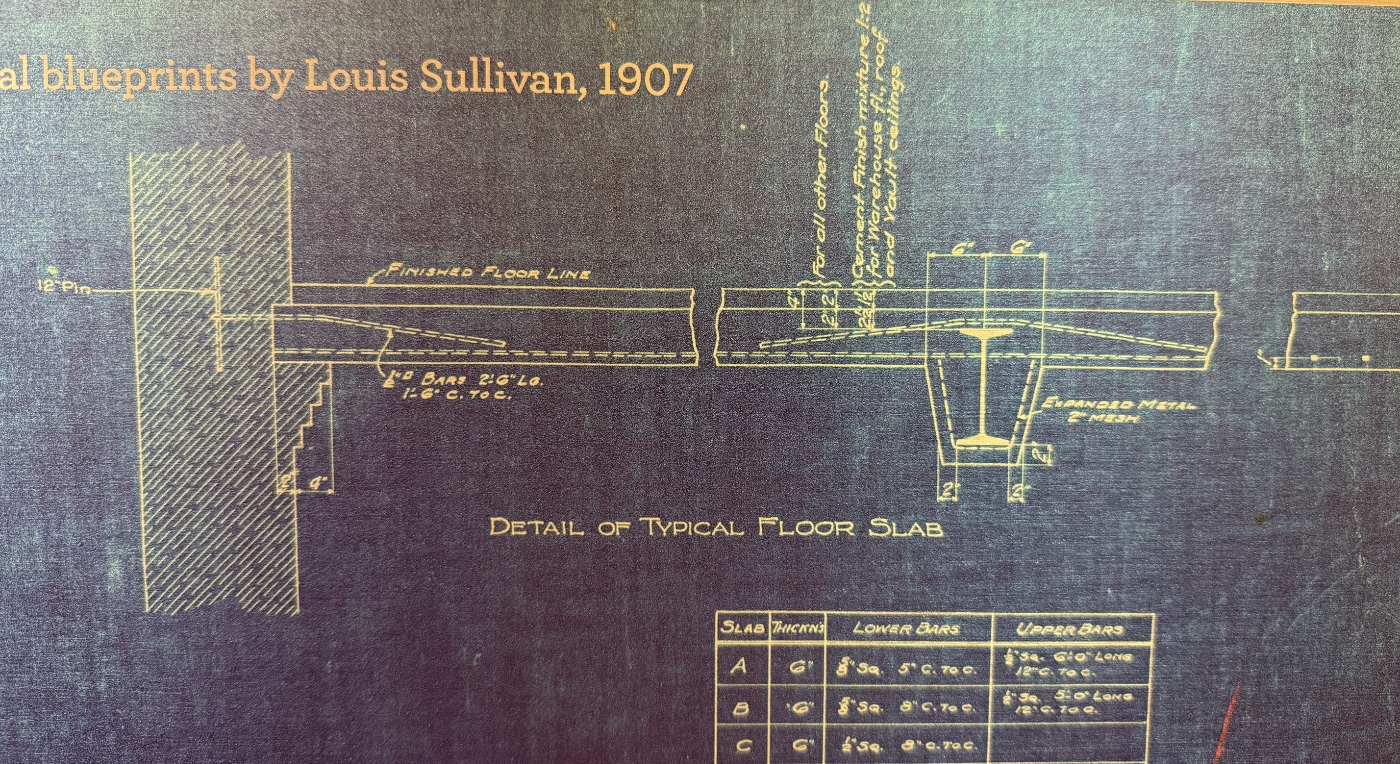
In an hallway above and behind the mezzanine, a few of Sullivan’s blueprints have been laminated and mounted on the walls.

As before, I got a closeup of the title block.

Also, there’s a wonderful detail of the floor slab. While reinforced concrete had been used well before this building, it still wasn’t especially common. What I like about this detail is that it isn’t very detailed. The theory of reinforced concrete was in its infancy, but Sullivan did seem to know that he needed top reinforcement in the negative moment areas.
If things go according to plan, I’ll be visiting the three jewel box banks in Iowa next week.
-
There are several other semicircular windows on the longer side of the building, but they’re not made of stained glass. ↩
Wisconsin jewel box bank
August 27, 2025 at 10:34 PM by Dr. Drang
You may recall my post from earlier this summer about the Louis Sullivan jewel box banks and how ChatGPT gave me the wrong information about the best way to tour all of them. Today I started a trip that should hit five of the eight banks. Oh, I’m also doing some other stuff, like visiting my kids.
The first bank on the trip is the one that ChatGPT left off its list entirely, the Farmers and Merchants Union Bank in Columbus, Wisconsin. The bank has had some sections added to it since the original construction in 1919; only the front and one side façade are still visible in full.


The semicircular stained glass windows are one of the building’s standout features, and they are especially beautiful from the inside. Here’s the front window,

the rear window (which you can’t see from the exterior because of the additions),

and the set of five side windows,

The rear window can be viewed up close because there’s a mezzanine next to it. Here’s a detailed view:

The bank has a little historical display on the mezzanine, and one of the tellers led me up to it so I could spend some time looking through its artifacts. There are examples of brick and terracotta from both the original 1919 construction and a major renovation in 1960.

The best thing on mezzanine, though, is a set of Sullivan’s blueprints for the bank. Here you can see the front and side elevations:

You can also see the source of my frustration in trying to photograph the blueprints—too much reflection off the glass that covers them. They’re easier to see in person. I did manage to get a halfway decent shot of Sullivan’s title block.

Here’s a nice view of several terracotta blocks in situ,

and an imposing eagle at the top of the rear façade,

The eagle is just visible over the top of the side wall.
I mentioned additions to the bank. The one in 1960 restored some of the damaged terracotta, which was nice. Not quite so nice was the extension off the back of the bank:

I guess you could be charitable and say the 1960 architect wanted to make sure you didn’t confuse his work with Sullivan’s.
Finally, here’s the plaque on the front façade commemorating the building’s designation as a national historic landmark:

Although this is the first jewel box bank on my tour, it’s the last one Sullivan designed. He went out with a bang.
Library database success story
August 22, 2025 at 10:34 PM by Dr. Drang
As I said back in June, one of my goals for my library search system (recently updated) was to be able to check on whether I already owned a book while in a used bookstore. I got to use it for that very purpose a couple of days ago, and it worked just as I hoped.
I saw a copy of Cornelius Lanczos’s The Variational Principles of Mechanics at a local used book store. In good shape and at a good price. This is a book I’ve known about for 40 years and one that’s very well known. It’s not the book that was assigned for the class I took on energy methods—that was Henry Langhaar’s Energy Methods in Applied Mechanics, a wonderful book that I’ve referred to throughout my career—but it was one that I’ve always thought I should own.
The problem was, as I stood there in the bookstore, that I couldn’t remember whether I’d ever followed through on that thought. Out came my phone. I brought up the search page, authenticated myself, and searched for “Lanczos.” Zero results. On the off-chance his name included some diacritical marks that weren’t on the book itself, I also searched for “Variational.” Still no hits, so I bought the book and added it to the system. Now it’s on my shelf and a search on his name returns this:

Would it have been a tragedy if I spent ten dollars on a second copy of a book I already owned? No. Did I spend more than ten dollars worth of effort building my library search system? Absolutely. But the satisfaction I got having the system work exactly as I’d hoped was priceless.
Fitness trends
August 19, 2025 at 5:12 PM by Dr. Drang
Last year, I complained about Apple’s way of calculating and presenting trends in the Health app. Today I’m going to complain about how it handles trends in the Fitness app. I like to spread my complaints around.
Here’s a screenshot I took this morning of the Trends section in Fitness:
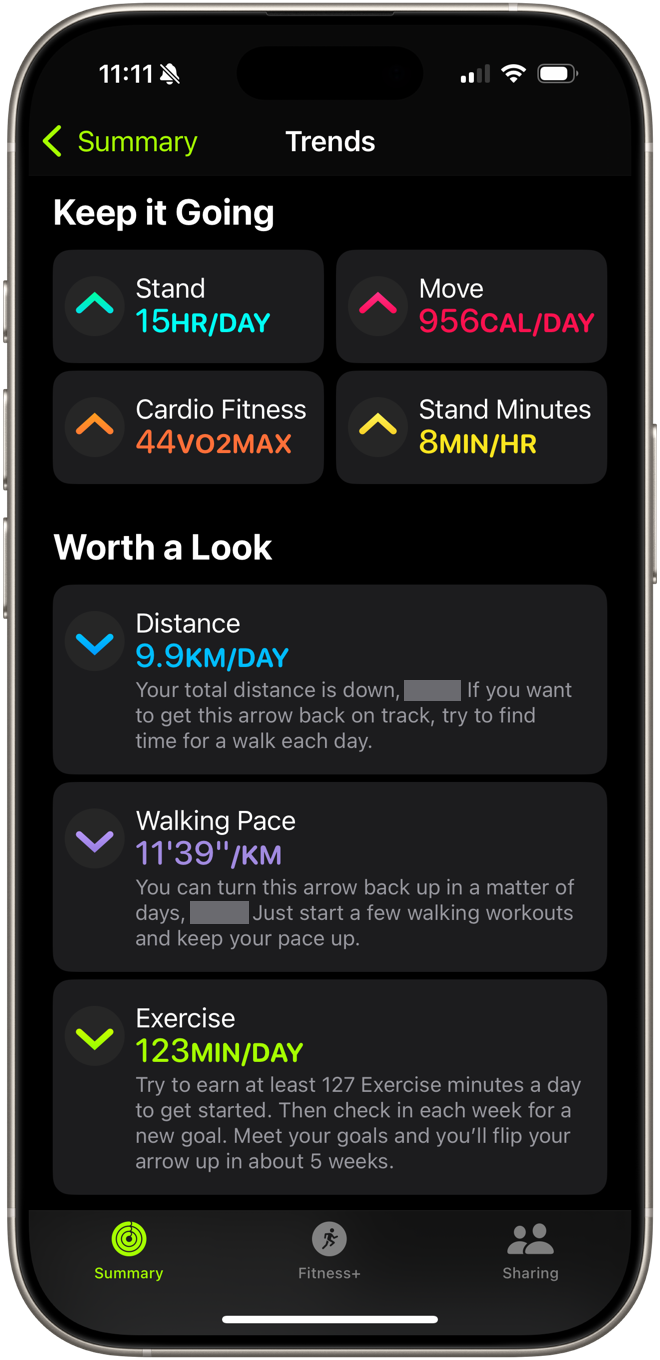
It’s happy with how much I’m standing, how many Move calories I’m burning, and my (calculated) cardio fitness, but it’s disappointed in the amount of time I spend exercising and my walking distance and pace. It gives me a little pep talk for each of these.
The thing is—and Fitness should know this because it has all the data—the reason I’ve been walking less the past few months is that I’ve gotten back into biking after several years away from it. And it’s reasonable that the time I spend on my bike is time that I can’t spend walking, isn’t it? Somehow, though, the Fitness app doesn’t see it that way.
You might raise an eyebrow at the lowered walking pace and exercise minutes. Why, you might ask, would riding a bike make those go down? Well, I get my heart rate higher when biking than when walking, so I tend to spend less time riding than walking. And when I do walk, it’s often a more casual exercise. A greater percentage of my walks now are hikes in the woods or trips to and from the store, and they go at a slower pace.
There’s also my twice-a-week kayaking, but that—and I don’t understand this at all—doesn’t seem to cut down on my walking credit. I know my step count increases as I paddle, which both the Fitness and Health apps treat as a kind of walking. That doesn’t happen when I’m on my bike. Maybe it’s because my arms are moving as I paddle but are still when I’m cycling, but honestly, when I tell Fitness I’m starting a paddling workout it ought to know I’m not walking.
We all know Apple keeps its programmers compartmentalized to reduce leaks. But this nonsense makes me think that programmers working on different parts of the same app are siloed. The alternative is that they can’t conceive of anyone doing different types of exercise at different times of the year.
When the weather turns cold in a few months, I assume Fitness will be happy to see me back walking instead of biking. Its praise then will be as meaningless as its disappointment is now.
