Next post Previous post

Graphical Pythagoras

July 3, 2010 at 9:47 PM by Dr. Drang

You may have seen this clever animated GIF floating around the intertubes lately. The original seems to live at http://i.imgur.com/DrROq.gif, but I have no idea who created it.

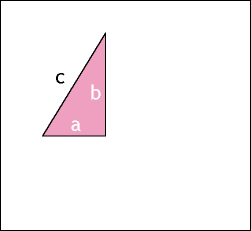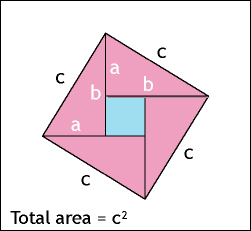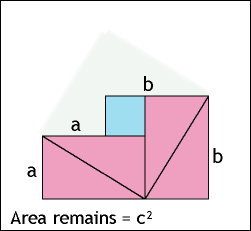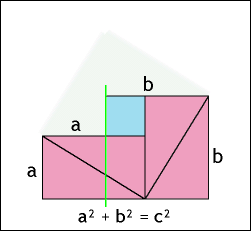
To me, the whole thing could have been done with just this frame.

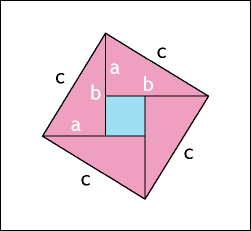
Each of the four pink triangles has an area of ba/2, the little blue square in the center has an area of (b−a) 2, and the big square formed by the hypotenuses has an area of c 2. So

4⋅(ba2)+(b−a) 2=c 2

Expanding the binomial gives us

2ba+(b 2−2ba+a 2)=c 2

The ba terms cancel, and we’re left with

a 2+b 2=c 2

as expected. It’s not as cute as the animation, but I like how the algebra works.

Next post Previous post